Returns the individual term binomial distribution probability. Use BINOMDIST in problems with a fixed number of tests or trials, when the outcomes of any trial are only success or failure, when trials are independent, and when the probability of success is constant throughout the experiment. For example, BINOMDIST can calculate the probability that two of the next three babies born are male.
Syntax
BINOMDIST(number_s,trials,probability_s,cumulative)
Number_s is the number of successes in trials.
Trials is the number of independent trials.
Probability_s is the probability of success on each trial.
Cumulative is a logical value that determines the form of the function. If cumulative is TRUE, then BINOMDIST returns the cumulative distribution function, which is the probability that there are at most number_s successes; if FALSE, it returns the probability mass function, which is the probability that there are exactly number_s successes.
Remarks
-
Number_s and trials are truncated to integers.
-
If number_s, trials, or probability_s is nonnumeric, BINOMDIST returns the #VALUE! error value.
-
If number_s < 0 or number_s > trials, BINOMDIST returns the #NUM! error value.
-
If probability_s < 0 or probability_s > 1, BINOMDIST returns the #NUM! error value.
-
The binomial probability mass function is:
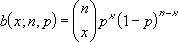
where:

is COMBIN(n,x).
Note: The COMBIN function is used here to illustrate the mathematical formula used by the BINOMDIST function. It is not a function that you can use in a list.
The cumulative binomial distribution is:
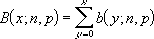
Example
|
Number_s |
Trials |
Probability_s |
Formula |
Description (Result) |
|
6 |
10 |
0.5 |
=BINOMDIST([number_s],[trials],[probability_s],FALSE) |
Probability of exactly 6 of 10 trials being successful (0.205078) |